4.3.3 Journal – Law of Sines and Proofs

Have you ever looked up at a majestic mountain, its peak piercing the sky, and wondered how its height could be calculated? Or perhaps you’ve observed the graceful flight of a bird, its path an elegant curve against the backdrop of the blue canvas. These scenarios, and many others, involve the fundamental concepts of trigonometry, where the Law of Sines plays a vital role. It’s a powerful tool that allows us to determine the lengths of sides and magnitudes of angles within triangles, whether it’s for mapping terrains or analyzing the motion of celestial bodies.

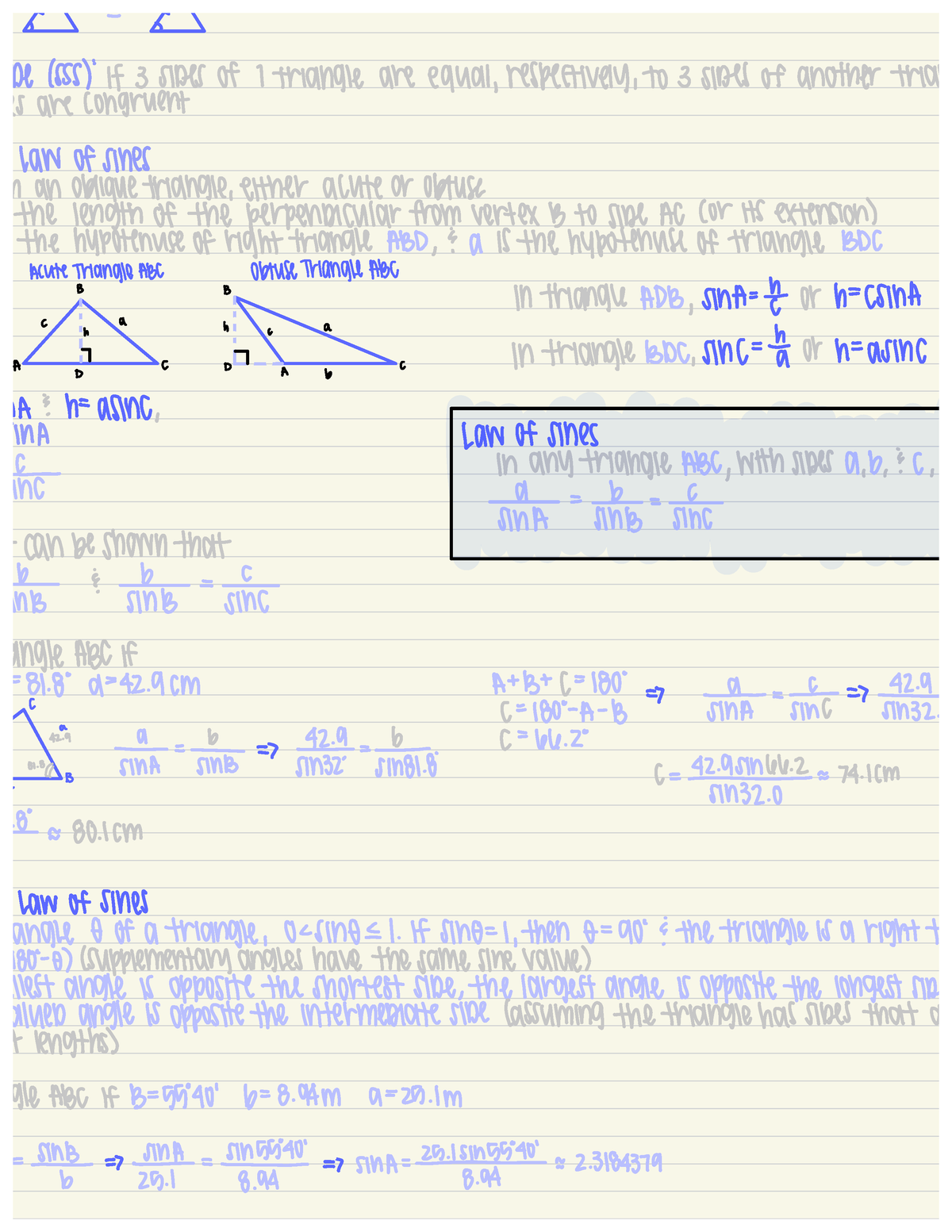
Image: www.studocu.com

This journal entry delves into the Law of Sines, exploring its theoretical foundation, practical applications, and proofs. We’ll unravel the intricacies of this trigonometric principle and equip you with the understanding to confidently navigate the world of triangles and their mysteries.

The Law of Sines: A Bridge Between Sides and Angles

The Law of Sines establishes a direct relationship between the sides and angles of any triangle. It asserts that the ratio of the length of a side to the sine of the angle opposite that side is constant for all three sides. In other words, if we denote the sides of a triangle as ‘a’, ‘b’, and ‘c’ and their corresponding opposite angles as ‘A’, ‘B’, and ‘C’, respectively, then the Law of Sines can be expressed as:

a/sin A = b/sin B = c/sin C

This elegant formula serves as a bridge, linking the geometric properties of a triangle’s sides and angles. Understanding the Law of Sines unlocks the ability to solve for unknown sides or angles when other information about the triangle is known.

Proofs of the Law of Sines: Unveiling the Logic

Proof Using the Area Formula

One approach to proving the Law of Sines involves utilizing the formula for the area of a triangle. We know that the area of a triangle can be calculated as half the product of two sides and the sine of the included angle. In a triangle ABC, we can express the area in three ways:

• Area = (1/2) * b * c * sin A
• Area = (1/2) * a * c * sin B
• Area = (1/2) * a * b * sin C

Equating these expressions for the area, we get:

• b * c * sin A = a * c * sin B
• a * c * sin B = a * b * sin C
• b * c * sin A = a * b * sin C

Simplifying these equations by dividing both sides by corresponding terms, we obtain the Law of Sines:

a/sin A = b/sin B = c/sin C

Image: www.coursehero.com

Proof Using the Unit Circle

A second method of proving the Law of Sines utilizes the unit circle. Consider a triangle ABC inscribed in a circle with radius ‘r’. Let the angle subtended by side ‘a’ at the center of the circle be ‘2A’. Using the sine function on the unit circle, we can express ‘sin A’ as (a / 2r). Similarly, ‘sin B’ = (b / 2r) and ‘sin C’ = (c / 2r). Since ‘r’ is a constant, we can eliminate it from each equation, yielding:

a / sin A = b / sin B = c / sin C

This method reinforces the relationship between the sine function and the unit circle, providing a visual perspective on the Law of Sines.

Applications of the Law of Sines: Solving Real-World Problems

The Law of Sines permeates various fields of study, offering solutions to seemingly complex problems. Some of its key applications include:

• Surveying and Mapping: Determining distances and angles between points on land.
• Navigation: Calculating distances and bearings for ships and aircraft.
• Astronomy: Analyzing the positions and motions of celestial bodies.
• Physics: Calculating forces and velocities in systems involving triangles.
• Engineering: Designing structures that are stable and efficient.

The Law of Sines serves as an essential tool for simplifying calculations and achieving precise solutions in these fields.

Tips for Applying the Law of Sines: Mastering the Technique

Accurately applying the Law of Sines requires a systematic approach and attention to detail. Here are some tips to enhance your understanding and problem-solving skills:

• Identify the Given information: Clearly identify the known sides and angles of the triangle.
• Choose the appropriate Formula: Select the version of the Law of Sines that incorporates the known and unknown quantities.
• Isolate the Unknown: Manipulate the formula to solve for the desired unknown side or angle.
• Verify Your Solution: Double-check your calculations and ensure that the solution makes sense in the context of the problem.

By following these steps, you can confidently navigate the world of triangles using the Law of Sines.

FAQs about the Law of Sines

Q: When is the Law of Sines applicable?

A: The Law of Sines is applicable to any triangle, provided you know at least one side and its opposite angle, or two angles and one side.

Q: How is the Law of Sines different from the Law of Cosines?

A: The Law of Cosines is used when you know two sides and the included angle or all three sides. The Law of Sines is used when you know one side and its opposite angle.

Q: Can I use the Law of Sines to solve for all angles and sides of a triangle?

A: While the Law of Sines helps to find many elements of a triangle, it might not be sufficient to find all angles and sides. In some cases, you might need to use the Law of Cosines or other trigonometric relationships.

4.3.3 Journal Law Of Sines And Proofs

Conclusion: Embracing the Power of Trig

The Law of Sines is a cornerstone of trigonometry, unlocking a wealth of possibilities for understanding the world around us. Its elegant formula connects sides and angles, providing a tool for solving problems in a range of scientific and engineering disciplines. By mastering this principle, you gain access to a powerful tool for making accurate calculations and comprehending the intricate relationships between geometric shapes.

Are you fascinated by triangles and the power of trigonometry? Please share your thoughts and questions in the comments below!