Unveiling the Mystery – Understanding the Power of Intermediate Algebra

Imagine standing at the brink of a vast, uncharted territory, a landscape filled with intricate patterns, perplexing equations, and hidden connections. This, my friend, is the world of intermediate algebra, a subject that often sparks both fascination and apprehension. Today, we embark on a journey to demystify this mathematical realm, revealing its power and relevance in shaping our world.

Image: math.stackexchange.com

Intermediate algebra, much like a skilled cartographer, provides the tools for understanding the intricate relationships behind the scenes of our world. It lays the foundation for countless disciplines, from engineering and economics to computer science and even music theory. But its true beauty lies in its ability to empower you, to see the invisible strings connecting seemingly disparate concepts and to make sense of the complex world around us. Let’s step into this world and uncover its secrets together.

A Bridge to Higher Mathematics: Understanding the Fundamentals

Intermediate algebra serves as a bridge between basic algebraic concepts and the complex world of calculus and advanced mathematics. You might recall the familiar equations from your first foray into algebra, the ones involving simple variables like “x” and “y.” Intermediate algebra takes those foundational concepts to the next level, introducing new powerful tools, building upon those initial equations, and unleashing their full potential.

The heart of intermediate algebra beats with the rhythm of functions. Functions are like mathematical machines, transforming inputs into outputs. They describe real-world relationships, like the connection between the number of hours you work and the amount of money you earn. In intermediate algebra, you’ll explore a vast array of function families, each with its unique traits: linear functions, quadratic functions, exponential functions, logarithmic functions, and more. Understanding how these functions work allows us to model complex phenomena, predict outcomes, and make informed decisions.

The Power of Exponents and Logarithms

One of the most significant milestones in our journey through intermediate algebra is delving into the world of exponents and logarithms. Exponents, as you might remember, are a shorthand for repeated multiplication. Think of 2 to the power of 3, or 2^3, which is simply 2 multiplied by itself three times (2 2 2 = 8). Logarithms, in turn, are the inverse of exponents. They answer the question: “To what power must we raise a base to get a certain number?”

This might sound complicated, but these powerful concepts lie at the heart of many technological advancements. From understanding compound interest growth in finance to calculating sound intensity in physics, the language of exponents and logarithms empowers us to unravel complex phenomena and unlock technological possibilities.

Solving Equations: Unveiling the Unknown

One of the primary goals of algebra, both basic and intermediate, is to solve equations. Equations are like puzzles, with a set of rules and a missing piece we need to find. In intermediate algebra, the puzzles become more intricate, often involving multiple variables, exponents, and logarithms. But with the right tools and techniques, we can crack the code and solve for our unknown variables.

This ability to solve equations unlocks potential in every field. Engineers use equations to design bridges and buildings, economists leverage them to model markets and allocate resources, and scientists employ them to analyze data and uncover the secrets of the universe. Each solution to an equation is a victory for knowledge and a step towards understanding the intricate workings of the world around us.

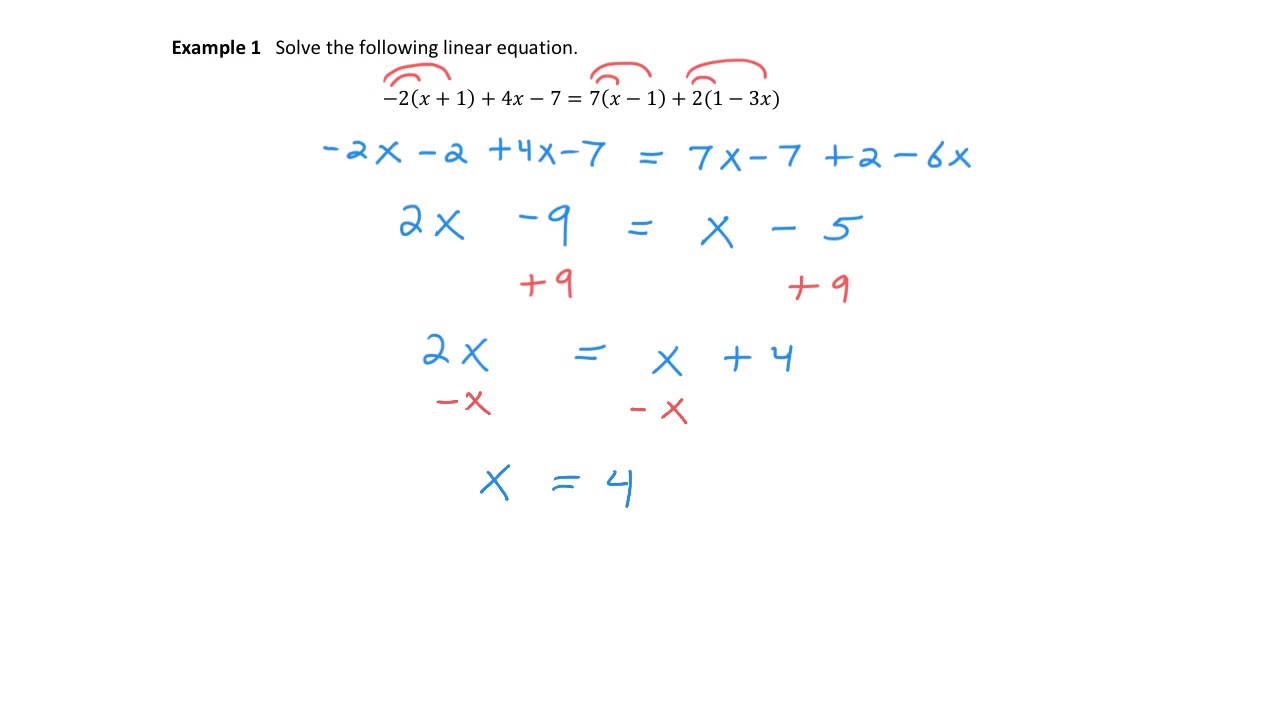
Image: www.youtube.com

A Window Into the Real World: The Power of Applications

The true magic of intermediate algebra lies in its ability to connect abstract concepts to the real world. We’ve already glimpsed how functions can represent tangible relationships, like the time it takes to travel a certain distance at a given speed. This same power applies to countless other scenarios.

For instance, exponential functions can model the growth of populations, the spread of diseases, or even the investment returns you might experience. Logarithmic functions, on the other hand, enable us to understand the decibel scale used to measure sound intensity, the Richter scale for earthquakes, and even the pH levels that determine the acidity or alkalinity of a substance.

Navigating the Challenges: Building Confidence and Mastering Skills

The path to mastering intermediate algebra might seem daunting at times. It requires a shift in thinking, a willingness to embrace new concepts, and a dedication to practice. But with the right approach and guidance, you can overcome these challenges and unlock the power of this remarkable subject.

Embracing the Learning Process:

• Don’t shy away from asking questions: Approach your instructor or tutor with any questions or areas of confusion. The more you ask, the more you learn.
• Practice, practice, practice: There’s no substitute for consistent practice. Work through examples, challenge yourself with practice problems, and seek out solutions when you encounter difficulties.
• Connect with fellow learners: Join study groups or online forums. Sharing ideas, helping each other, and exchanging insights can significantly enhance your understanding.
• Seek out resources: Explore libraries, online platforms, and educational videos for supplementary materials and explanations tailored to your learning style.

Mastering the Essentials:

• Strengthen your fundamental algebra skills: Review basic algebraic manipulations, solving equations, and working with fractions. A solid foundation is crucial for success in intermediate algebra.
• Focus on understanding the concepts: Don’t just memorize formulas, strive to understand the underlying logic and reasoning behind them. This will help you apply those concepts in new and unfamiliar contexts.
• Visualize the problems: Use diagrams, graphs, and real-world scenarios to represent equations and functions. This can make a significant difference in understanding abstract concepts.

Beyond the Classroom: A Gateway to Opportunities

The doors that open when you master intermediate algebra are numerous and exciting. It paves the way for further studies in mathematics, statistics, and countless STEM fields. But it also equips you with valuable skills that can be applied in everyday life, from making informed financial decisions to analyzing data and solving complex problems in various settings.

Intermediate Algebra Meaning

A Word of Encouragement: Embrace the Journey

The path to unlocking the power of intermediate algebra might not always be smooth sailing. But, remember, every challenge you conquer, every concept you grasp, brings you closer to a deeper understanding of the world around you. It is a journey worth taking, one that will empower you to see the world through the eyes of a mathematician, to decipher the intricate patterns woven into the fabric of our universe, and to harness the potential of this powerful subject for a deeper and more fulfilling life.

So, take that first step. Pick up a textbook, explore online resources, or find a tutor. Embrace the journey, and watch as the world of intermediate algebra unfolds before you, revealing a hidden world of beauty, interconnectedness, and endless possibilities.