Angular Kinematic Equations – Mastering Rotational Motion

Imagine a child spinning a top. It’s a simple, yet fascinating example of angular motion. As the top whirls, its speed changes, and it even begins to tilt and wobble. This seemingly straightforward action is governed by a set of equations known as angular kinematic equations. These equations provide a powerful framework for understanding and predicting the motion of objects rotating about an axis, from spinning tops to planets revolving around the sun.

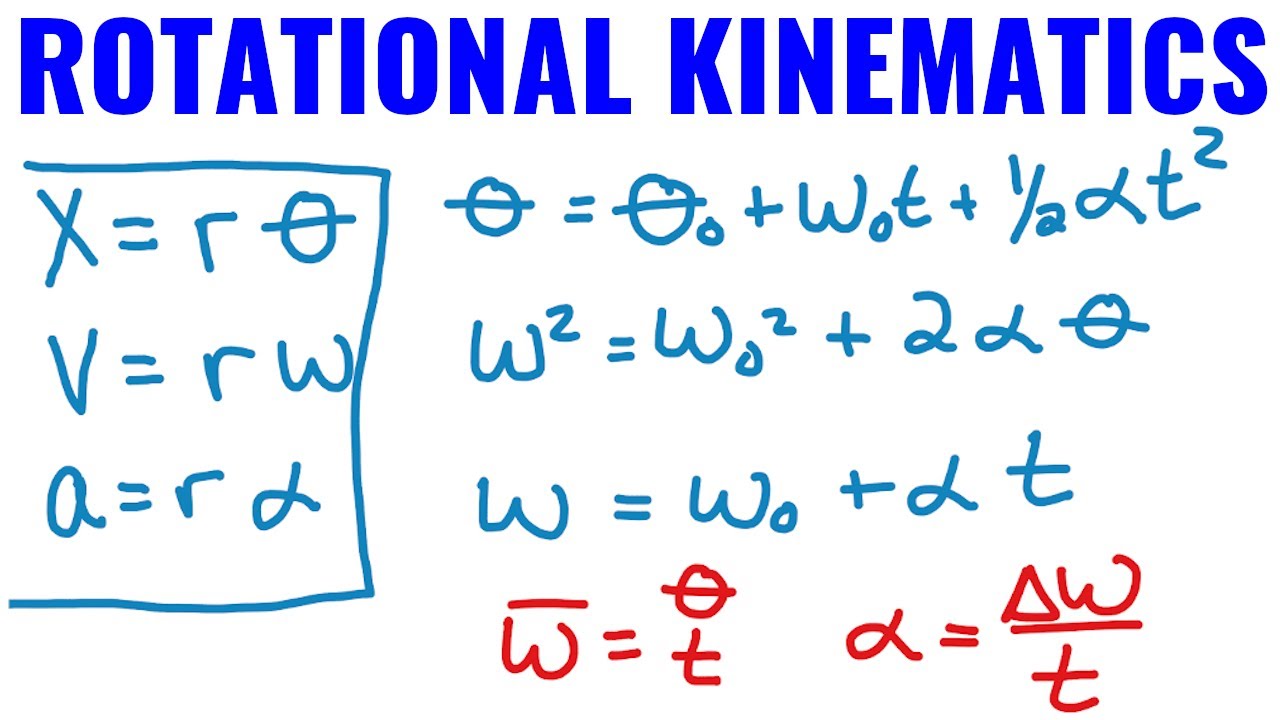
Image: materialcampuselois.z13.web.core.windows.net

These equations are not just for theoretical physics. They have practical applications in fields like engineering, robotics, and even sports. From designing efficient motors to optimizing the spin of a baseball, understanding angular kinematic equations can be instrumental in achieving optimal performance.

Exploring Angular Kinematic Equations

Understanding the Fundamentals

Angular kinematics deals with the motion of objects rotating about a fixed axis. Unlike linear kinematics, which focuses on straight-line motion, angular kinematics explores rotations, twists, and spins. The key quantities involved are angular displacement, angular velocity, and angular acceleration.

Angular displacement refers to the change in angle of an object. It is measured in radians, and its symbol is θ. Angular velocity, denoted by ω, is the rate of change of angular displacement over time. It is measured in radians per second (rad/s). Finally, angular acceleration, symbolized by α, describes the rate of change of angular velocity over time. Its unit is radians per second squared (rad/s²).

The Equations of Angular Motion

Five fundamental equations govern angular motion:

1. ω = ω_o + αt (Describes the final angular velocity (ω) in terms of initial angular velocity (ω_o), angular acceleration (α), and time (t)).
2. θ = ω_ot + (1/2)αt² (Relates angular displacement (θ) to initial angular velocity, angular acceleration, and time).
3. ω² = ω_o² + 2αθ (Connects final angular velocity to initial angular velocity, angular acceleration, and angular displacement).
4. θ = (ω + ω_o)t / 2 (Expresses angular displacement as the average of initial and final angular velocities multiplied by time).
5. θ = ω_ot + (1/2)αt² (Relates angular displacement (θ) to initial angular velocity, angular acceleration, and time).

These equations provide a powerful tool for analyzing and predicting the behavior of rotating objects. By applying these equations, we can determine the angular displacement, velocity, and acceleration of an object at any given time, allowing for precise control and understanding of rotational motion.

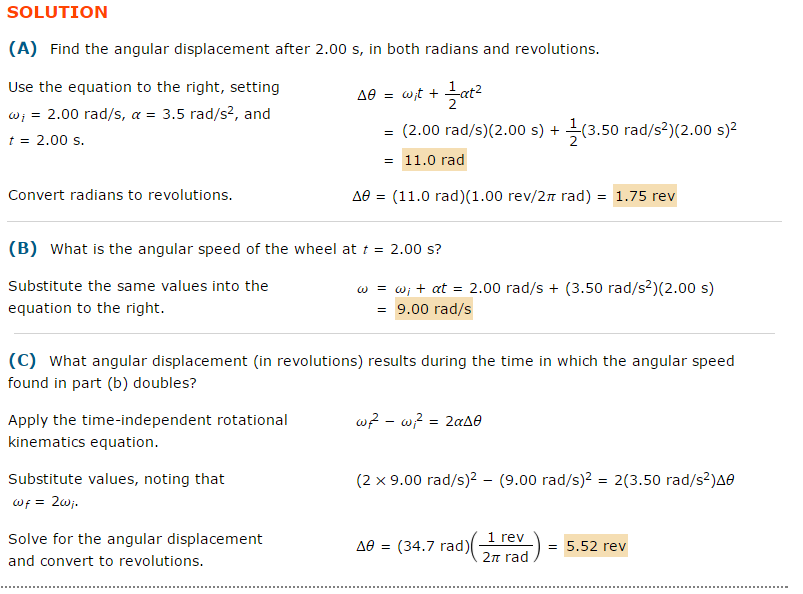
Image: www.chegg.com

Key Concepts in Angular Kinematics

In addition to the core equations, understanding several other crucial concepts is essential for applying angular kinematics effectively.

• Torque: This is the rotational equivalent of force. Just as force causes linear acceleration, torque causes angular acceleration. It is defined as the product of the force applied and the distance from the axis of rotation.
• Moment of Inertia: This is a measure of an object’s resistance to changes in its angular velocity. It’s similar to mass in linear motion. The larger the moment of inertia, the more difficult it is to rotate the object.
• Angular Momentum: This quantity represents the mass in motion of a rotating object. It is analogous to linear momentum and is calculated as the product of the object’s moment of inertia and its angular velocity.

Understanding these concepts alongside the angular kinematic equations provides a comprehensive framework for analyzing and comprehending rotational motion.

Recent Advancements in Angular Kinematics

The field of angular kinematics is constantly evolving, with new research and applications emerging regularly. Recent advancements are particularly prominent in the area of nanotechnology.

Engineers are utilizing angular motion principles to develop nanoscale devices, particularly in areas like microfluidics. For example, microfluidic chips that rely on rotating components for precise fluid manipulation are being actively developed for use in medical diagnostics, drug delivery, and other biomedical applications.

Additionally, the field of robotics is witnessing significant progress in leveraging angular kinematic equations to create sophisticated robots capable of complex motions. These robots are being designed for applications such as surgery, manufacturing, and exploration of hazardous environments. The advancements in both fields highlight the ongoing relevance and potential of angular kinematic equations.

Tips for Understanding Angular Kinematics

Mastering angular kinematics isn’t always easy, but with the right approach, it can become a manageable and rewarding endeavor. Here are some tips to assist you:

• Visualize the Motion: Try to visualize the rotational motion of the object. Imagine the direction of rotation, the location of the axis of rotation, and how the object’s motion might change over time. This can help you understand the concepts more intuitively.
• Use Analogies: Linear motion concepts can be a helpful entry point. Try to draw parallels between angular displacement and linear displacement, angular velocity and linear velocity, and so on.
• Practice Problem-Solving: Apply the angular kinematic equations to various problems. Start with simpler examples and gradually work your way up to more challenging scenarios. By tackling a variety of problems, you’ll reinforce your understanding and gain greater confidence.

Expert Advice: Bridging the Gap

Many students find it challenging to bridge the gap between theoretical concepts and real-world applications of angular kinematic equations. One effective approach is to focus on the physical interpretations of the equations. Instead of simply memorizing formulas, try to understand what each equation tells about the object’s motion and how it relates to everyday scenarios.

For example, the equation ω = ω_o + αt describes the change in angular velocity over time. So, you can visualize this as an object accelerating or decelerating as it rotates. Apply this understanding to specific examples like a spinning wheel or a rotating fan. By connecting the equations to real-world phenomena, you will develop a deeper appreciation for their practical significance.

FAQ on Angular Kinematic Equations

1. What is the difference between angular velocity and angular speed?

Angular velocity is a vector quantity, possessing both magnitude and direction. It represents both how fast an object is rotating and the direction of its rotation. Meanwhile, angular speed is a scalar quantity, representing only the rate of rotation.

2. Can angular acceleration be negative?

Yes, angular acceleration can be negative. It indicates that the angular velocity is decreasing. A negative acceleration implies that the object is slowing down its rotation.

3. How does torque relate to angular acceleration?

Torque is the force that causes angular acceleration. Similar to how force causes linear acceleration, torque causes a change in an object’s rotational motion. The relationship between torque and angular acceleration is given by the equation τ = Iα, where τ is the torque, I is the moment of inertia, and α is the angular acceleration.

Angular Kinematic Equations

Conclusion

Understanding angular kinematic equations is crucial for grasping the fundamentals of rotational motion. These equations provide a powerful framework for analyzing and predicting the behavior of rotating objects, with diverse applications in various fields. By mastering these equations and applying the provided tips, you can gain a deeper understanding of this fundamental aspect of physics and its real-world implications.

Are you interested in diving deeper into angular kinematic equations and exploring its applications? Let us know your thoughts and questions in the comments below!