Prime Factorization of 75 – A Deep Dive

Remember those long division problems from elementary school? While they might seem like a distant memory, the concepts we learned back then are still relevant in our daily lives, particularly in the realm of mathematics. One such concept is prime factorization, which plays a crucial role in understanding the building blocks of numbers. Today, we’ll embark on a journey to uncover the prime factors of 75, delving into the process and its significance.

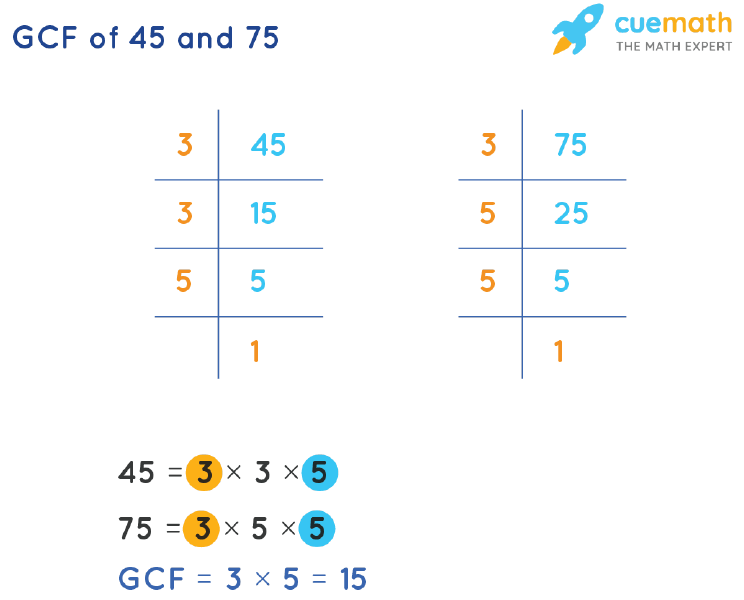
Image: www.cuemath.com

Imagine you’re baking a cake and need to split your ingredients into equal portions. Prime factorization can help you determine the most efficient way to divide your ingredients. Similarly, in mathematics, understanding the prime factors of a number can unlock insights into its properties and relationships with other numbers.

Understanding Prime Factorization

Prime factorization is a fundamental concept in number theory that involves breaking down a composite number into its prime factors. A prime factor is a prime number that divides the original number without leaving a remainder. To clarify, a prime number is a whole number greater than 1 that has only two divisors: 1 and itself.

For example, consider the number 12. It is a composite number because it has more than two divisors (1, 2, 3, 4, 6, and 12). The prime factorization of 12 is 2 x 2 x 3, where 2 and 3 are prime numbers. These numbers, when multiplied together, result in the original number, 12.

Methods for Prime Factorization

1. Factor Tree Method:

The factor tree method is a visually appealing way to determine the prime factors of a number. It starts by branching out the original number into two factors. Then, you continue branching out each factor until you arrive at prime numbers.

For instance, let’s factorize 75:

• 75
• 3 x 25
• 3 x 5 x 5

Therefore, the prime factorization of 75 is 3 x 5 x 5 or 3 x 5².

Image: findthefactors.com

2. Division Method:

The division method involves repeatedly dividing the original number by prime numbers until you arrive at a prime number. The prime numbers used in the division process are the prime factors of the original number.

To factorize 75 using the division method:

• 75 ÷ 3 = 25
• 25 ÷ 5 = 5
• 5 ÷ 5 = 1

The prime factors used in the division are 3 and 5. Therefore, the prime factorization of 75 is 3 x 5 x 5 or 3 x 5².

Importance of Prime Factorization

Prime factorization, though seemingly a simple concept, has numerous applications in various mathematical fields and everyday scenarios. Here are some significant uses:

• Finding the Greatest Common Factor (GCD): Prime factorization makes it easy to find the GCD of two or more numbers. The GCD is the largest number that divides two or more numbers without leaving a remainder. To find the GCD, you simply multiply the common prime factors of the numbers, raised to their lowest powers.
• Finding the Least Common Multiple (LCM): The LCM is the smallest number that is a multiple of two or more numbers. To find the LCM, multiply all the prime factors of the numbers, including the common ones, raised to their highest powers.
• Simplifying Fractions: Prime factorization can help simplify fractions by finding common factors in the numerator and denominator. By canceling out these common factors, you obtain a simplified fraction.
• Cryptography: Prime factorization plays a crucial role in cryptography, a field dedicated to secure communication. RSA (Rivest-Shamir-Adleman) encryption, commonly used to protect online transactions, relies on the difficulty of factoring large composite numbers into their prime factors.
• Number Theory: Prime factorization is a fundamental tool in number theory, a branch of mathematics that deals with the properties of integers. It is used to prove theorems and solve problems related to divisibility, remainders, and other integer properties.

Prime Factorization in the Real World

Beyond mathematics, prime factorization finds applications in everyday situations. Imagine you’re organizing a party and have to divide guests into groups. Prime factorization can help you determine the most efficient way to divide the guests into equal-sized groups. In this case, understanding the prime factors of the total number of guests will help you ensure that each group has the same number of guests.

Tips and Expert Advice

Mastering prime factorization can be a valuable skill. Here are some tips and advice to help you become a factorization pro:

• Start with the smallest prime number, 2: When factorizing a number, always start by checking if it is divisible by 2. If it is, divide it by 2 and continue factoring the result.
• Use divisibility rules: Divisibility rules can help you quickly determine if a number is divisible by a specific prime number. For instance, if a number ends in 0 or 5, it is divisible by 5. If the sum of the digits of a number is divisible by 3, the number is also divisible by 3.
• Practice, practice, practice: Like any skill, prime factorization becomes easier with practice. Try factorizing different numbers, gradually increasing their complexity. Don’t be afraid to use a calculator or online tools to help you along the way.
• Visualize the process: Using factor trees or a division method can help you visualize the process of prime factorization and make it easier to understand.
• Explore further: Once you grasp the basics of prime factorization, explore its applications in different fields. Learn how it is used in cryptography, number theory, and other areas.

Prime Factorization: An Essential Mathematical Tool

Prime factorization is an essential mathematical tool that allows us to break down numbers into their fundamental building blocks. Understanding prime factors opens the door to a deeper understanding of number theory, cryptography, and everyday problems. From dividing guests at a party to simplifying fractions, prime factorization is a valuable skill that can be applied across various domains.

FAQ

Q: What is the prime factorization of 75?

A: The prime factorization of 75 is 3 x 5 x 5 or 3 x 5².

Q: Why is prime factorization important?

A: Prime factorization is crucial as it allows us to break down numbers into simpler components, aiding in various mathematical operations like finding the GCD, LCM, simplifying fractions, and playing a critical role in cryptography and number theory.

Q: How can I learn more about prime factorization?

A: You can explore various online resources like educational websites, Khan Academy videos, and mathematics textbooks dedicated to number theory for a deeper dive into prime factorization and its applications.

Prime Factorization Of 75

Conclusion

Understanding prime factorization is essential for anyone interested in mathematics and its applications. From breaking down numbers into prime factors to comprehending their implications in everyday situations, this mathematical concept holds significant value. Remember, practice makes perfect, so don’t hesitate to delve into the world of prime factorization and explore its fascinating aspects.

Are you interested in learning more about prime factorization or its applications in different fields?