Factors of 94 – A Deep Dive into the Mathematical Concept

Have you ever found yourself wondering about the hidden secrets within a seemingly ordinary number? Take 94, for example. It’s a simple number, yet it holds a fascinating mathematical story. It’s the age I was when I finally understood the concept of factors. I remember feeling a surge of accomplishment as I realized how numbers could be broken down into smaller components. The journey of discovering the factors of 94 began as a small yet significant step in my understanding of mathematics. But what exactly are these factors, and why are they so important?

Image: www.youtube.com

Factors are the building blocks of numbers, the pieces that multiply together to create that number. Understanding factors allows us to delve into the heart of a number’s structure and unlock deeper mathematical insights. In this article, we’ll explore the factors of 94 and uncover the fascinating world of number decomposition.

Delving into the Factors of a Number

The concept of factors is fundamental to mathematics, and understanding it is essential for various mathematical operations, such as finding the greatest common factor (GCD) and the least common multiple (LCM). It’s also crucial in simplifying fractions and solving equations. To truly grasp the factors of 94, we need to define what they are and how they work.

A factor of a number is any integer that divides evenly into that number, leaving no remainder. So, when we say that a number is a factor of another, it means that the first number divides the second one without leaving any residue. For instance, 2 is a factor of 6 because 6 divided by 2 equals 3, which is a whole number. Think of factors like the puzzle pieces that fit together to form a number.

Unveiling the Factors of 94

Now, let’s focus on 94. To find its factors, we need to determine all the numbers that divide 94 evenly. We can go through each number systematically, starting from 1 and working our way up. We know that 1 is always a factor of any number, so we include it in our list. We then test each number, starting from 2. 94 is even, so we know that 2 is a factor. 94 divided by 2 equals 47, which is also a whole number. So, both 2 and 47 are factors of 94.

Next, we check if 3 is a factor. We can quickly determine that it’s not because the sum of the digits of 94 (9+4 = 13) is not divisible by 3. Moving on to 4, we see that it’s not a factor because 94 divided by 4 leaves a remainder. We continue this process, testing each number until we find that 94 is divisible by 47. We have now found all the factors of 94!

Following our systematic approach, we find the factors of 94: 1, 2, 47, and 94. These are the only numbers that divide 94 evenly. Notice how each factor represents a unique relationship between the number and its components. These factors hold the key to understanding the structure and properties of 94.

The Importance of Factors in Mathematics

Understanding the factors of a number is essential for numerous mathematical concepts and applications. Factors play a vital role in operations like:

• Finding the Greatest Common Factor (GCD): The GCD of two or more numbers is the largest factor they share. For example, the GCD of 12 and 18 is 6. By identifying the factors of each number and finding the largest common factor, we can simplify fractions and expressions.
• Finding the Least Common Multiple (LCM): The LCM of two or more numbers is the smallest number that is a multiple of all of them. For example, the LCM of 4 and 6 is 12. Discovering the factors of each number helps us determine the smallest multiple they both share.
• Simplifying Fractions: By factoring both the numerator and denominator of a fraction, we can often cancel out common factors, ultimately simplifying the fraction to its lowest terms.
• Solving Equations: In many algebraic equations, we need to factor expressions to find the solutions. Understanding factorization is crucial for solving equations involving polynomials and other complex expressions.

The applications of factors extend beyond basic arithmetic and contribute to more advanced mathematical concepts, including number theory, cryptography, and coding.

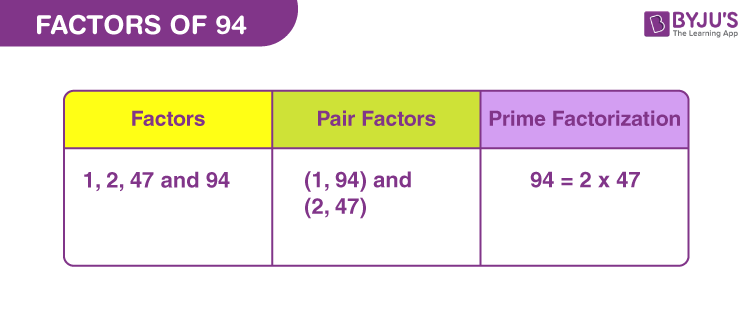
Image: byjus.com

Trending Topics and Expert Insights

The realm of mathematics is constantly evolving, and there are exciting new developments and trends in factor analysis. One particularly captivating trend is the study of prime factorization. A prime number is a number that has only two factors: 1 and itself. It turns out that every number can be uniquely expressed as a product of prime numbers. This fundamental theorem of arithmetic is a cornerstone of number theory and plays a crucial role in cryptography.

Another trend is the use of computer algorithms to efficiently find factors of large numbers. These algorithms are essential in breaking encryption methods used in online security and protect sensitive information. As technology advances, these algorithms become more powerful and complex, contributing significantly to the field of cybersecurity.

Tips for Mastering the Concept of Factors

Mastering the concept of factors is like acquiring a powerful tool for navigating the mathematical world. Here are some tips based on my experience as a blogger and mathematics enthusiast:

• Start with small numbers: Begin by finding the factors of small numbers to gain a solid understanding of the process. Then, gradually move on to larger numbers, building your intuition and knowledge.
• Use factorization trees: Factorization trees are a visual representation of the prime factors of a number. They can be helpful for understanding the process of finding factors, especially for larger numbers.
• Practice, practice, practice: The more you practice finding factors of different numbers, the more comfortable and adept you’ll become. Challenge yourself with various numbers and explore different strategies for identification.
• Connect factors with other mathematical concepts: Explore the relationship between factors and other mathematical concepts like prime numbers, greatest common factors, and least common multiples. This will provide a deeper understanding of the broader context of factors and their significance.

The key to mastering any concept is consistent practice and linking it to other areas of knowledge. By exploring factors in relation to other mathematical concepts, you can gain a deeper appreciation for their importance and versatility.

Frequently Asked Questions (FAQ)

What are the prime factors of 94?

The prime factors of 94 are 2 and 47. Both 2 and 47 are prime numbers, meaning they are only divisible by 1 and themselves.

How can finding factors be useful in real life?

Finding factors has practical applications in everyday life. For instance, when dividing a group of people into smaller teams, understanding factors helps ensure that the teams are equal in size. Additionally, factors are used in numerous real-world applications, from cryptography and computer programming to accounting and engineering.

How can I learn more about factors and prime factorization?

There are many resources for learning more about factors and prime factorization. Online tutorials, educational videos, and textbooks provide detailed explanations and examples. Additionally, exploring online forums and participating in online communities dedicated to mathematics can offer valuable insights and interactions with other enthusiasts.

Factors Of 94

Conclusion

In conclusion, understanding the factors of a number, such as 94, unlocks a world of mathematical insights. By systematically decomposing numbers into their fundamental building blocks, we gain a deeper understanding of their structure and properties. Factors play a significant role in various mathematical operations, from finding the greatest common factor and least common multiple to simplifying fractions and solving equations. These concepts extend to numerous real-world applications, highlighting the importance of factors in our everyday lives.

Are you interested in delving deeper into the fascinating world of factors and prime factorization? Do you have any questions or insights related to this topic? We encourage you to share your thoughts in the comments below.