03125 as a Fraction – Unraveling the Mystery of Decimals and Fractions

Have you ever encountered a decimal number like 0.3125 and wondered how to express it as a fraction? The world of numbers is full of fascinating connections, and one of the most intriguing is the relationship between decimals and fractions. This seemingly simple conversion can unveil a deeper understanding of how we represent numbers and their underlying power.

Image: brainly.in

This article delves into the transformation of 0.3125 into its fractional form. We will explore the fundamental concepts of decimals and fractions, uncover the process of converting decimals to fractions, and see how this knowledge can be applied in various real-world scenarios.

Understanding Decimals and Fractions

Before we embark on the conversion journey, let’s clarify the essence of decimals and fractions.

• Decimals: A decimal number represents a part of a whole number using the base-10 system. Each digit to the right of the decimal point holds a value that is a power of 10. For example, in 0.3125, the “3” represents 3 tenths, the “1” represents 1 hundredth, the “2” represents 2 thousandths, and the “5” represents 5 ten-thousandths.

• Fractions: A fraction represents a part of a whole where the whole is divided into equal parts. The top number (numerator) shows how many parts are being considered, and the bottom number (denominator) indicates the total number of equal parts in the whole. For instance, 1/2 represents one out of two equal parts of a whole.

Converting 0.3125 to a Fraction

Now, let’s convert 0.3125 into a fraction. Here’s the process:

1. Identify the Decimal Place: The decimal 0.3125 has four digits after the decimal point, which means it represents ten-thousandths (1/10000).

2. Write as a Fraction: We can write 0.3125 as 3125/10000.

3. Simplify the Fraction: Both the numerator and denominator are divisible by 5. We can simplify the fraction by dividing both by 5: 625/2000. We can simplify further by dividing both by 5 again: 125/400. This can be simplified one more time, dividing both by 25: 5/16.

Therefore, 0.3125 is equivalent to the fraction 5/16.

Real-World Applications

The ability to convert decimals to fractions has numerous practical applications in various fields:

• Measurements: In engineering and construction, measurements are often expressed as fractions. Converting decimals to fractions ensures accurate calculations and precise measurements.

• Cooking and Baking: Recipes frequently call for ingredients measured in fractions. Knowing how to convert decimals to fractions allows for accurate ingredient proportions.

• Finance: In financial calculations, decimals and fractions are used to represent percentages, interest rates, and stock prices. This knowledge is essential for understanding financial statements and making informed financial decisions.

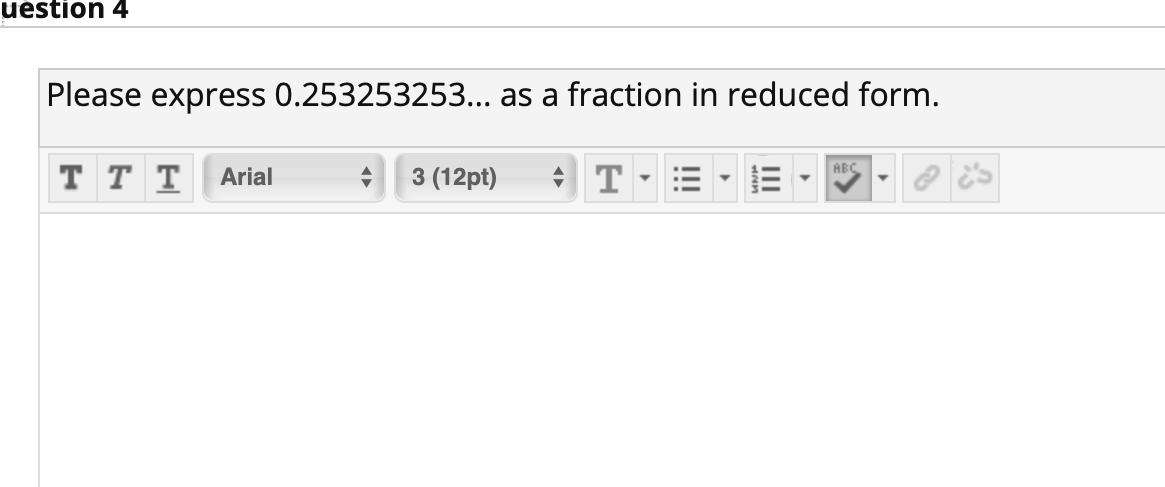
Image: www.chegg.com

Beyond the Basics: Exploring Different Decimal Forms

While 0.3125 is a simple example, decimals can take on various forms. Understanding how to convert different types of decimals to fractions is crucial for comprehensive knowledge of this concept.

• Terminating Decimals: Decimals like 0.3125, which have a finite number of digits after the decimal point, are called terminating decimals. They can easily be converted to fractions as demonstrated above.

• Repeating Decimals: Decimals like 0.3333… (where the 3 repeats infinitely) are called repeating decimals. They require a slightly different conversion process. To convert a repeating decimal to a fraction, follow these steps:

  1. Set up an Equation: Let ‘x’ equal the repeating decimal. For example, x = 0.3333…

  2. Multiply to Shift the Decimal: Multiply both sides of the equation by 10 (or 100, 1000, etc., depending on the number of repeating digits) to shift the decimal. In this case, 10x = 3.3333…

  3. Subtract the Original Equation: Subtract the original equation (x = 0.3333…) from the multiplied equation (10x = 3.3333…). This results in 9x = 3.

  4. Solve for x: Divide both sides by 9 to find x: x = 3/9, which can be simplified to 1/3.

  Therefore, 0.3333… is equivalent to the fraction 1/3.

03125 As A Fraction

Conclusion

Understanding the intricacies of converting decimals to fractions is a valuable skill for navigating various mathematical and real-world scenarios. This knowledge empowers you to express numbers in different ways, enhancing your analytical abilities and problem-solving skills. Whether it’s calculating measurements, following recipes, or understanding financial statements, the ability to seamlessly convert between decimals and fractions proves its worth. The journey from 0.3125 to 5/16 is a testament to the interconnectedness of the mathematical world, reminding us that numbers hold a powerful and fascinating story waiting to be unveiled.